|
 |
- Search
| J. Electromagn. Eng. Sci > Volume 19(2); 2019 > Article |
|
Abstract
In this paper, we propose a compact-standard antenna method (C-SAM) for antenna calibration above 1 GHz. The test-site evaluation of the fully-anechoic room (FAR) condition satisfied the free-space conditions. When the C-SAM was compared with conventional antenna calibration methods, the maximum deviation was within ┬▒0.18 dB for the 1ŌĆō18 GHz frequency range. Unlike the conventional antenna calibration methods, the proposed method is a simple standard antenna method that calculates the antenna factor of the antenna under calibration (AUC) with only one site insertion loss (SIL) measurement of an antenna calibration site that meets free-space conditions. Therefore, the C-SAM is the best candidate for antenna calibration owing to the methodŌĆÖs simplicity and cost-reduction potential.
The recent rapid development of information and communication technology (ICT) has increased the development and utilization of ICT equipment. This increase has created a complex propagation environment, which has expanded the electromagnetic interference (EMI) problem. Internationally, to distribute electric and electronic equipment on the market, companies must obtain electromagnetic compatibility (EMC) certification [1, 2].
The core equipment for EMC certification is an antenna that measures EMI. If this antenna is damaged, the inherent propagation characteristics of the antenna are also changed. Therefore, it is not possible to provide accurate EMC certification results.
For this reason, the importance of the calibration method for an EMI antenna to measure its inherent propagation characteristics is increasing. Calibrating an antenna for EMI measurements involves measuring the antenna factor (AF), which means the conversion coefficient of the measured voltage and the electric field strength.
As shown in Table 1, the typical antenna calibration methods above 1 GHz specified in the international standard CISPR 16-1-6 [3] include the three-antenna method (TAM) [4, 5] and the standard antenna method (SAM) [6]. The TAM is based on the Friis equation. This method calculates the AF of the antenna under calibration (AUC) using three antennas with no previous knowledge of the AF. The SAM does not consider the ground reflected wave. In this condition, the AF of the AUC is calculated with two measurements through the difference between the site insertion loss (SIL) of the AUC and the SIL of the standard antenna (STA) from the transmitting antennas for which previous knowledge is not known. These methods must perform SIL measurements two or three times by using the three antennas to calculate the AF. Setting up these measurements involves high cost and a long measurement time to calibrate the antenna.
Many studies have been carried out to deal with the limitations of conventional calibration methods [7ŌĆō10]. These studies showed that the AF of the AUC can be calculated with a single measurement if the AF is already known using antennas with the same characteristics. However, the antenna calibration method is only for low frequencies (30 MHz to 1 GHz) using a diode loaded standard dipole antenna [7, 8].
The antenna calibration methods presented by Lim et al. [9, 10] are restricted to frequency bands ranging from 3.95ŌĆō5.85 GHz and 26.5ŌĆō40 GHz, respectively. Moreover, there is no test-site evaluation method in which the antenna calibration test site can be proven to be free space [10].
In this paper, we propose a compact-standard antenna method (C-SAM) for a broad frequency range from 1 GHz to 18 GHz which is different from conventional antenna methods. Unlike the TAM, the C-SAM has the advantage of knowing the AF of the AUC with one measurement. In addition, the C-SAM differs from the SAM, because the ground reflected wave is considered. In other words, if the AF is known for one antenna, this method can calculate the AF of the AUC with only one SIL measurement.
To use this method above 1 GHz, the antenna calibration test site must meet free-space conditions. For this reason, the test site was verified using the test site evaluation of the fully-anechoic room (FAR) condition. In addition, the C-SAM was verified by comparing it with conventional antenna calibration methods (the SAM and the TAM).
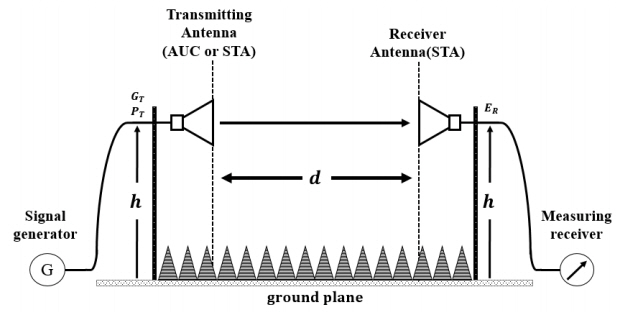
As shown in Fig. 1, the C-SAM is an antenna calibration method that fixes the distance (d) and height (h) between the STA and the AUC. Then, the AF of the AUC can be calculated with the SIL measurement between two antennas, where the STA is an antenna whose AF is already known. The pyramidal horn antenna is used for antenna calibration above 1 GHz.
The C-SAM is based on the Friis equation [11]. As shown in Fig. 1, if information about the electric field strength (E
R
) is given at the receiving location, then the distance d
1 between the transmitting antenna and the receiving antenna is as follows:
where G
T
is the gain of the transmitting antenna, and P
T
is the output power of the transmitting antenna.
AF is the parameter that determines the unique performance of the antenna. AF is defined as the ratio of the field strength (E) to the voltage (V) induced at the receiving antenna as follows:
When the transmitting antenna (T
X
) AF is AF
TX
, and the receiving antenna (R
X
) AF is AF
RX
, AF can be calculated as follows:
SIL is a site insertion loss between the two antennas, f
MHz
is the frequency in MHz, and d is the separation distance in meters.
If the STA that knows the values either AF
RX
or AF
TX
is used in Eq. (3), then the AF of the AUC can be calculated as only one measurement with the following equation:
AF
STA
is the AF of the STA, AF
AUC
is the AF of the AUC, SIL
STA
is the site insertion loss of the STA, and SIL
AUC
is the site insertion loss of the AUC. Now, AF
AUC
and AF
STA
must be calculated at the same position (the antenna height (h) and the separation distance (d) from R
X
antenna). In other words, if there is an STA whose AF is known, then the C-SAM can easily calculate the AF of the AUC with only one measurement.
Eq. (3) is based on the Friis equation. Thus, the C-SAM should be applied in the FAR condition test site. The aim is to create a free-space environment for calibrating antennas. This method is applied to calibrate antennas with directivity above 1 GHz.
In other words, the C-SAM is an antenna calibration method above 1 GHz and is based on the Friis equation.
Therefore, before verifying this method, the antenna calibration test site must prove that it is free space. The verification method for the FAR condition is defined in detail in CISPR 16-1-5.
As shown in Fig. 2, the measurement configuration of the test-site evaluation method of the FAR condition is that the broadband horn antennas (T
X
and R
X
) are placed at a height of 2 m from the ground plane. The antennas used for the measurement are the Schwarzbeck BBHA 9120 D model. The direction of the two antennas is vertical polarization, and an absorber is placed on the ground plane. The measurement method calculates the SIL between the antennas by moving the distance of the T
X
antenna from the fixed R
X
antenna to 2.8 m, 2.9 m, 3.0 m, 3.1 m, and 3.2 m. The measurement frequency range is 1ŌĆō18 GHz (500 MHz steps).
The calculated distance relative to the SIL for FAR validation via the measurement results is as follows:
where S
21cable
is the transmission cable loss ratio, and S
21antennas
is the transmission loss ratio of the antennas. A
im
(d) for each varied distance by the movement T
X
antenna is normalized for 3 m, which is the central position of the T
X
antenna, can be defined as the following equation:
If the maximum and minimum deviations of the A
im
(d)
Normalized 3m
for each distance are within ┬▒0.5 dB (peak to peak A
im
(d) Ōēż ┬▒0.5 dB), the test site is said to satisfy the FAR condition [12].
The result for the test-site evaluation was within ┬▒0.5 dB (peak to peak A
im
(d) Ōēż ┬▒0.5 dB), in the range of 1ŌĆō18 GHz (Fig. 3). Therefore, the antenna calibration test site may be defined as free-space conditions for the frequency range 1ŌĆō18 GHz.
The measurement configuration of the C-SAM is shown in Fig. 4, where the distance between the two antennas (d = 3 m), the antenna height (h = 2 m), and the absorbers are installed on the ground plane.
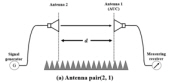
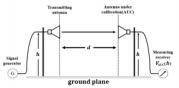
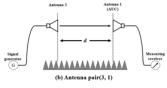
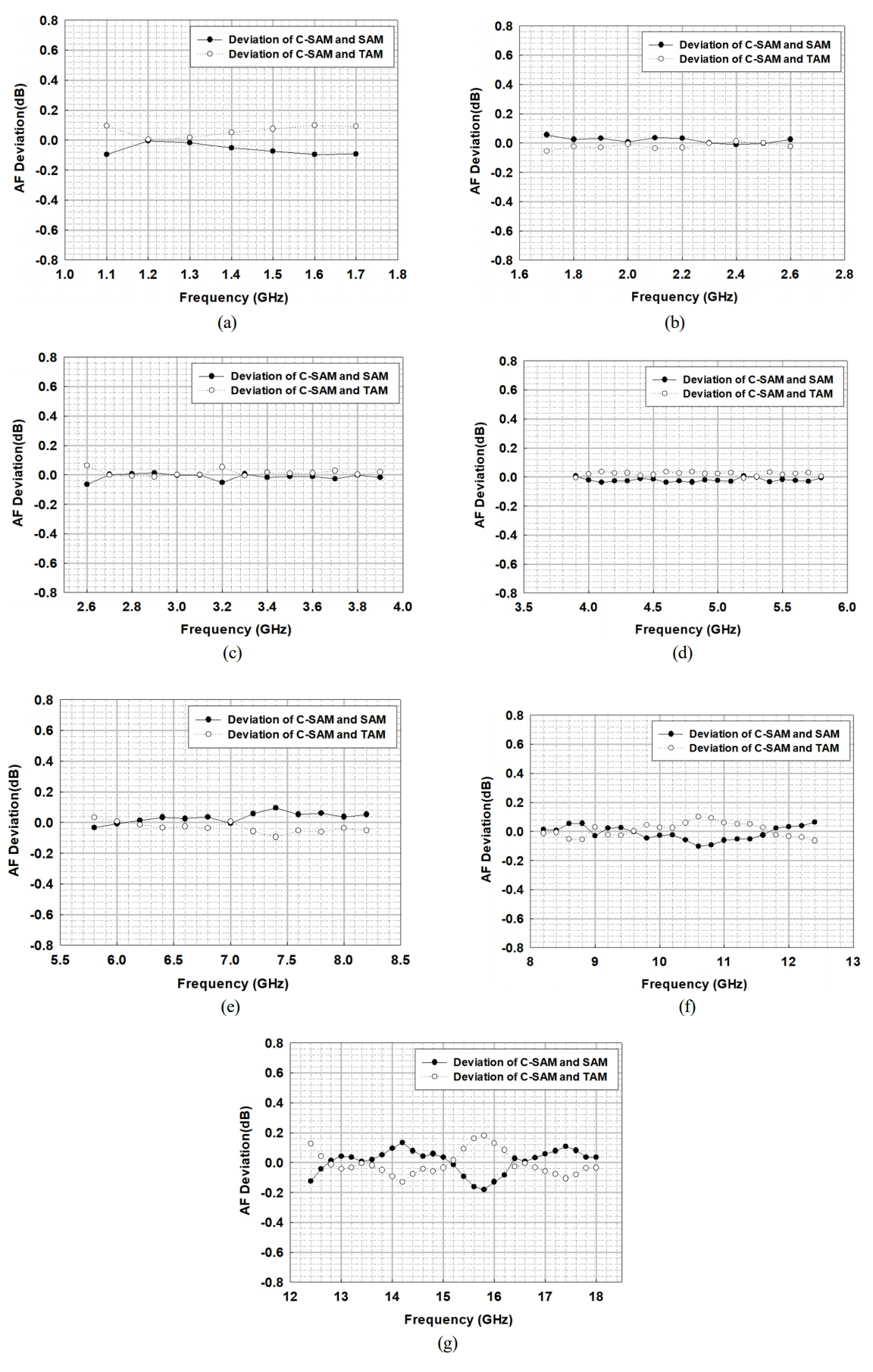
The validation method for the C-SAM is as follows. The AUC (1ŌĆō18 GHz broadband horn antenna C) measures the SIL using the C-SAM, TAM, and SAM, and the AF. The two antennas except the AUC are pyramidal horn antennas. For this reason, each measurement frequency band is divided into 7 sub-bands (1.12ŌĆō1.70 GHz, 1.7ŌĆō2.6 GHz, 2.60ŌĆō3.95 GHz, 3.95ŌĆō5.85 GHz, 5.85ŌĆō8.20 GHz, 8.20ŌĆō12.4 GHz, and 12.4ŌĆō 18.0 GHz). Then the AFs calculated by the C-SAM and the conventional methods were compared. The measurement configuration and the AF calculation method of the TAM and the SAM are shown in Table 1.
The AFs of the wideband antenna (C) obtained with the three methods are compared in Fig. 5. The maximum deviation was found to be within ┬▒0.18 dB, which was recorded at 15.8 GHz. There are small deviations from the conventional methods and compared results. The C-SAM uses the AF calculated using Eq. (3). In addition, the C-SAM is a calibration method that measures the AF of the AUC at one time with the measurement configuration shown in Table 1. Therefore, the measurement configuration such as the distance between the antennas and the height is very important. A deviation may occur due to errors in the measurement setup. Thus, the experimental results verify that C-SAM can be used for antenna calibration for the wide frequency range of 1ŌĆō18 GHz.
In this study, we proposed a C-SAM antenna calibration method above 1 GHz. To apply this method, we performed a test-site evaluation of the FAR condition to determine whether it was free space. The maximum and minimum deviations of the A
im
(d)
Normalized 3m
for each distance were within ┬▒0.5 dB, and the test site was validated as the free-space condition. The C-SAM was compared with conventional antenna calibration methods at the test site satisfying the FAR condition, and the proposed method was verified by confirming that the maximum deviation for 1ŌĆō18 GHz was ┬▒0.18 dB. Contrary to conventional antenna calibration schemes, and if one AF is known on the calibration test site that satisfies the free-space condition, the number of SIL measurements can be reduced using the C-SAM. In addition, this method can be a suitable candidate for the revision of the measure CISPR 16-1-6 and a reduction in the measurement cost.
Acknowledgments
This work was supported by the ICT R&D program of MSIT/IITP (No. 2019-0-00102, A study on public health and safety in a complex EMF environment).
Fig.┬Ā5
The AF for the C-SAM, TAM, and SAM: (a) 1.12ŌĆō1.70 GHz, (b) 1.7ŌĆō2.6 GHz, (c) 2.60ŌĆō3.95 GHz, (d) 3.95ŌĆō5.85 GHz, (e) 5.85ŌĆō 8.20 GHz, (f) 8.20ŌĆō12.4 GHz, and (g) 12.4ŌĆō18.0 GHz.
Table┬Ā1
Comparison of conventional antenna calibration methods and the C-SAM
References
1. S Lee, K Lee, and W Nah, "Reduction of conducted emission in interleaved RPWM buck converter," The Journal of Korean Institute of Electromagnetic Engineering and Science, vol. 28, no. 4, pp. 298ŌĆō308, 2017.
2. TH Hubing, "Performance-based EMC design using a maximum radiated emissions calculator," Journal of Electromagnetic Engineering and Science, vol. 13, no. 4, pp. 199ŌĆō207, 2013.

4. KT Selvan, "Preliminary examination of a modified three-antenna gain measurement method to simplify uncertainty estimation," IEEE Antennas and Propagation Magazine, vol. 45, no. 2, pp. 78ŌĆō81, 2003.

5. KT Selvan, "A modified three-antenna gain measurement method to simplify uncertainty estimation," Progress In Electromagnetics Research, vol. 57, pp. 197ŌĆō208, 2006.

6. N Suzuki, A Sugiura, Y Yamanaka, and T Iwasaki, "Calibration of biconical antennas using the standard antenna method," In: Proceedings of 1999 International Symposium on Electromagnetic Compatibility; Tokyo, Japan. 1999, pp 588ŌĆō591.

7. J Park, G Mun, D Yu, B Lee, and WN Kim, "Proposal of simple reference antenna method for EMI antenna calibration," In: Proceedings of 2011 IEEE International Symposium on Electromagnetic Compatibility; Long Beach, CA. 2011, pp 90ŌĆō95.

8. J Park, WN Kim, BG Kang, and H Yeon, "A novel reference antenna method for EMI antenna calibration," In: Proceedings of 2014 31st URSI General Assembly and Scientific Symposium (URSI GASS); Beijing, China. 2014, pp 1ŌĆō4.

9. JH Lim, BW Lee, SH Park, YJ Choi, and MW Seo, "A study of standard antenna method using two homogeneous horn antennas," In: Proceedings of 2016 URSI Asia-Pacific Radio Science Conference (URSI AP-RASC); Seoul, Korea. 2016, pp 793ŌĆō795.

10. JH Lim, BW Lee, YJ Choi, and HB Kim, "A study of measuring a commercial antenna gain using an R-SAM," In: Proceedings of 2017 Asia-Pacific International Symposium on Electromagnetic Compatibility (APEMC); Seoul, Korea. 2017, pp 131ŌĆō133.

11. HT Friis, "A note on a simple transmission formula," Proceedings of the IRE, vol. 34, no. 5, pp. 254ŌĆō256, 1946.
http://doi.org/10.1109/JRPROC.1946.234568
Biography
Min-Joo Jeong received the B.S. degree in Electronics Engineering from Chosun University, Korea, in 2013 and the M.S. degree in LED Fusion Engineering from Pukyong National University, Korea, in 2015. He is currently pursuing the Ph.D. degree in Information and Communication Engineering at Chungbuk National University, Cheongju, Korea. His research interests include antenna calibration, EMC measurements, antenna design, and EMF.

Biography
Jong-Hyuk Lim received the B.S. degree from Hongik University, Korea, in 2004 and the M.S. and Ph.D. degrees in Electronics Engineering from Hanyang University, Seoul, Korea, in 2006 and 2012, respectively. He is currently working at National Radio Research Agency (RRA), Naju, Korea. His research interests include antenna calibration, EMC measurements, millimeter-wave propagation modeling, THz communications, and rainfall attenuation.

Biography
Ji-Woong Park received the B.S. degree in Electronics Engineering from Chungbuk National University, Korea, in 2017. He is currently pursuing the M.S. degree in Information and Communication Engineering at Chungbuk National University, Cheongju, Korea. His research interests include antenna design, EMC, and EMF.

Biography
Nam Kim received the B.S., M.S., and Ph.D. degrees in Electronics Engineering from Yonsei University, Seoul, Korea, in 1981, 1983, and 1988, respectively. Dr. Kim is a Member of the International Advisory Committee for the World Health Organization project on EMF, the IEEE International Committee on Electromagnetic Safety, and the International Electro Technical Commission TC 106, and he was the President of the Bioelectromagnetics Society. He has been a Professor in the School of Information and Communication Engineering, Chungbuk National University, Cheongju, Korea, since 1989. His scientific interests are focused on the health effect of the EMF, RF dosimetry and SAR, reduction and protection technology of the EMF hazards, and guidelines and standards for the EMF.

Biography
Sung-Won Park received the M.S. degree in Information and Communication Engineering from Kyung Hee University, Seoul, Korea, in 2003. He is currently working at National Radio Research Agency (RRA), Naju, Korea. His research interests include antenna calibration, EMC measurements, millimeter-wave propagation modeling, THz communications, and rainfall attenuation.

- TOOLS
- Related articles in JEES
-
Compact Size Wideband Microstrip Antenna Element for Repeater and Base Stations at 2 GHz2001 ;1(1)